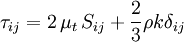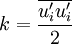Two equation turbulence models
From CFD-Wiki
(→Boussinesq eddy viscosity assumption: included k term in eqn for reynolds stress for incomp flows) |
|||
| Line 10: | Line 10: | ||
The basis for all two equation models is the [[Boussinesq eddy viscosity assumption]], which postulates that the [[Reynolds stress tensor]], <math>\tau_{ij}</math>, is proportional to the mean strain rate tensor, <math>S_{ij}</math>, and can be written in the following way: | The basis for all two equation models is the [[Boussinesq eddy viscosity assumption]], which postulates that the [[Reynolds stress tensor]], <math>\tau_{ij}</math>, is proportional to the mean strain rate tensor, <math>S_{ij}</math>, and can be written in the following way: | ||
| - | :<math>\tau_{ij} = 2 \, \mu_t \, S_{ij}</math> | + | :<math>\tau_{ij} = 2 \, \mu_t \, S_{ij} + \frac{2}{3}\rho k \delta_{ij}</math> |
| - | Where <math>\mu_t</math> is a scalar property called the [[Eddy viscosity|eddy viscosity]]. The | + | Where <math>\mu_t</math> is a scalar property called the [[Eddy viscosity|eddy viscosity]] which is normally computed from the two transported variables. The last term is included for modelling incompressible flow to ensure that the definition of turbulence kinetic energy is obeyed: |
| - | :<math> | + | :<math>k=\frac{\overline{u'_i u'_i}}{2}</math> |
| - | + | The same equation can be written more explicitly as: | |
| + | |||
| + | :<math> -\overline{\rho u'_i u'_j} = \mu_t \, \left( \frac{\partial U_i}{\partial x_j} + \frac{\partial U_j}{\partial x_i} \right) + \frac{2}{3}\rho k \delta_{ij}</math> | ||
The Boussinesq assumption is both the strength and the weakness of two equation models. This assumption is a huge simplification which allows one to think of the effect of turbulence on the mean flow in the same way as molecular viscosity affects a laminar flow. The assumption also makes it possible to introduce intuitive scalar turbulence variables like the turbulent energy and dissipation and to relate these variables to even more intuitive variables like [[Turbulence intensity|turbulence intensity]] and [[Turbulence length scale|turbulence length scale]]. | The Boussinesq assumption is both the strength and the weakness of two equation models. This assumption is a huge simplification which allows one to think of the effect of turbulence on the mean flow in the same way as molecular viscosity affects a laminar flow. The assumption also makes it possible to introduce intuitive scalar turbulence variables like the turbulent energy and dissipation and to relate these variables to even more intuitive variables like [[Turbulence intensity|turbulence intensity]] and [[Turbulence length scale|turbulence length scale]]. | ||
Revision as of 12:37, 13 June 2007
Two equation turbulence models are one of the most common type of turbulence models. Models like the k-epsilon model and the k-omega model have become industry standard models and are commonly used for most types of engineering problems. Two equation turbulence models are also very much still an active area of research and new refined two-equation models are still being developed.
By definition, two equation models include two extra transport equations to represent the turbulent properties of the flow. This allows a two equation model to account for history effects like convection and diffusion of turbulent energy.
Most often one of the transported variables is the turbulent kinetic energy, 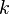 . The second transported variable varies depending on what type of two-equation model it is. Common choices are the turbulent dissipation,
. The second transported variable varies depending on what type of two-equation model it is. Common choices are the turbulent dissipation,  , or the specific dissipation,
, or the specific dissipation,  . The second variable can be thought of as the variable that determines the scale of the turbulence (length-scale or time-scale), whereas the first variable,
. The second variable can be thought of as the variable that determines the scale of the turbulence (length-scale or time-scale), whereas the first variable, 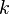 , determines the energy in the turbulence.
, determines the energy in the turbulence.
Boussinesq eddy viscosity assumption
The basis for all two equation models is the Boussinesq eddy viscosity assumption, which postulates that the Reynolds stress tensor, 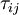 , is proportional to the mean strain rate tensor,
, is proportional to the mean strain rate tensor, 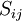 , and can be written in the following way:
, and can be written in the following way:
Where 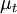 is a scalar property called the eddy viscosity which is normally computed from the two transported variables. The last term is included for modelling incompressible flow to ensure that the definition of turbulence kinetic energy is obeyed:
is a scalar property called the eddy viscosity which is normally computed from the two transported variables. The last term is included for modelling incompressible flow to ensure that the definition of turbulence kinetic energy is obeyed:
The same equation can be written more explicitly as:
The Boussinesq assumption is both the strength and the weakness of two equation models. This assumption is a huge simplification which allows one to think of the effect of turbulence on the mean flow in the same way as molecular viscosity affects a laminar flow. The assumption also makes it possible to introduce intuitive scalar turbulence variables like the turbulent energy and dissipation and to relate these variables to even more intuitive variables like turbulence intensity and turbulence length scale.
The weakness of the Boussinesq assumption is that it is not in general valid. There is nothing which says that the Reynolds stress tensor must be proportional to the strain rate tensor. It is true in simple flows like straight boundary layers and wakes, but in complex flows, like flows with strong curvature, or strongly accelerated or decellerated flows the Boussinesq assumption is simply not valid. This give two equation models inherent problems to predict strongly rotating flows and other flows where curvature effects are significant. Two equation models also often have problems to predict strongly decellerated flows like stagnation flows.

 model
model
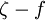 model
model