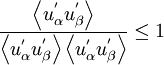Realisability and Schwarz' inequality

From CFD-Wiki

Revision as of 12:27, 12 June 2007 (view source) Si (Talk | contribs) (New page: Realisability is the minimum requirement to prevent a turbulence model generating non-physical results. For a model to be realisable the normal Reynolds stresses must be non-negative and t...) ← Older edit		Revision as of 12:32, 12 June 2007 (view source) Si (Talk | contribs) Newer edit →
Line 1:		Line 1:
-	Realisability is the minimum requirement to prevent a turbulence model generating non-physical results. For a model to be realisable the normal Reynolds stresses must be non-negative and the Schwarz' inequality must be satisfied between fluctuating quantities:	+	{{Turbulence modeling}}Realisability is the minimum requirement to prevent a turbulence model generating non-physical results. For a model to be realisable the normal Reynolds stresses must be non-negative and the Schwarz' inequality must be satisfied between fluctuating quantities:
	:<math>		:<math>

---

Revision as of 12:32, 12 June 2007

Turbulence modeling

Turbulence

RANS-based turbulence models Linear eddy viscosity models Algebraic models Cebeci-Smith model Baldwin-Lomax model Johnson-King model A roughness-dependent model One equation models Prandtl's one-equation model Baldwin-Barth model Spalart-Allmaras model Two equation models k-epsilon models Standard k-epsilon model Realisable k-epsilon model RNG k-epsilon model Near-wall treatment k-omega models Wilcox's k-omega model Wilcox's modified k-omega model SST k-omega model Near-wall treatment Realisability issues Kato-Launder modification Durbin's realizability constraint Yap correction Realisability and Schwarz' inequality Nonlinear eddy viscosity models Explicit nonlinear constitutive relation Cubic k-epsilon EARSM v2-f models model model Reynolds stress model (RSM)

Large eddy simulation (LES) Smagorinsky-Lilly model Dynamic subgrid-scale model RNG-LES model Wall-adapting local eddy-viscosity (WALE) model Kinetic energy subgrid-scale model Near-wall treatment for LES models

Detached eddy simulation (DES)

Direct numerical simulation (DNS)

Turbulence near-wall modeling

Turbulence free-stream boundary conditions Turbulence intensity Turbulence length scale

Realisability is the minimum requirement to prevent a turbulence model generating non-physical results. For a model to be realisable the normal Reynolds stresses must be non-negative and the Schwarz' inequality must be satisfied between fluctuating quantities:

where there is no summation over the indices. Some workers only apply the first inequality to satisfy realisability, or maintain non-negative vales of k and epsilon. This "weak" form of realisability is satisfied in non-linear models by setting .

References

Speziale, C.G. (1991), "Analytical methods for the development of Reynolds-stress closures in turbulence", Ann. Rev. Fluid Mechanics, Vol. 23, pp107-157.