Explicit nonlinear constitutive relation
From CFD-Wiki
(Difference between revisions)
| Line 1: | Line 1: | ||
{{Template: Turbulence modeling}} | {{Template: Turbulence modeling}} | ||
| + | |||
| + | == General Concept == | ||
An explicit nonlinear constitutive relation for the Reynolds stresses represents an explicitly-postulated expansion over the [[Linear eddy viscosity models|linear Boussinesq hypothesis]]. | An explicit nonlinear constitutive relation for the Reynolds stresses represents an explicitly-postulated expansion over the [[Linear eddy viscosity models|linear Boussinesq hypothesis]]. | ||
| Line 28: | Line 30: | ||
Note that the terms in the first line are exactly the linear relation as expressed by the Boussinesq hypothesis. | Note that the terms in the first line are exactly the linear relation as expressed by the Boussinesq hypothesis. | ||
| - | + | == Reference == | |
* {{reference-paper|author=Wallin, S., and Johansson, A. V.|year=2000|title=An Explicit Algebraic Reynolds Stress Model for Incompressible and Compressible Turbulent Flows|rest=Journal of Fluid Mechanics, Vol. 403, Jan. 2000, pp. 89–132}} | * {{reference-paper|author=Wallin, S., and Johansson, A. V.|year=2000|title=An Explicit Algebraic Reynolds Stress Model for Incompressible and Compressible Turbulent Flows|rest=Journal of Fluid Mechanics, Vol. 403, Jan. 2000, pp. 89–132}} | ||
{{stub}} | {{stub}} | ||
Latest revision as of 20:15, 4 November 2009
General Concept
An explicit nonlinear constitutive relation for the Reynolds stresses represents an explicitly-postulated expansion over the linear Boussinesq hypothesis.
One of such explicit and nonlinear expansion over the Boussinesq hypothesis, as proposed by [Wallin & Johansson (2000)], is given by

Note that the terms in the first line are exactly the linear relation as expressed by the Boussinesq hypothesis.
Reference
- Wallin, S., and Johansson, A. V. (2000), "An Explicit Algebraic Reynolds Stress Model for Incompressible and Compressible Turbulent Flows", Journal of Fluid Mechanics, Vol. 403, Jan. 2000, pp. 89–132.

 model
model
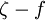 model
model