Linear eddy viscosity models
From CFD-Wiki
(Difference between revisions)
m |
m |
||
| Line 15: | Line 15: | ||
This linear relationship is also known as ''the Boussinesq hypothesis''. For a deep discussion on this linear constitutive relationship, check section [[Introduction to turbulence/Reynolds averaged equations]]. | This linear relationship is also known as ''the Boussinesq hypothesis''. For a deep discussion on this linear constitutive relationship, check section [[Introduction to turbulence/Reynolds averaged equations]]. | ||
| - | There are several subcategories for the linear eddy-viscosity models, depending on the number of (transport) equations | + | There are several subcategories for the linear eddy-viscosity models, depending on the number of (transport) equations solved for to compute the eddy viscosity coefficient. |
# [[Algebraic turbulence models|Algebraic models]] | # [[Algebraic turbulence models|Algebraic models]] | ||
Revision as of 22:29, 30 October 2009
These are turbulence models in which the Reynolds stresses, as obtained from a Reynolds averaging of the Navier-Stokes equations, are modelled by a linear constitutive relationship with the mean flow straining field, such as:
where 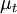 is the coefficient termed turbulence "viscosity" (also called the eddy viscosity), and
is the coefficient termed turbulence "viscosity" (also called the eddy viscosity), and 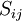 is the mean strain rate defined by:
is the mean strain rate defined by:
This linear relationship is also known as the Boussinesq hypothesis. For a deep discussion on this linear constitutive relationship, check section Introduction to turbulence/Reynolds averaged equations.
There are several subcategories for the linear eddy-viscosity models, depending on the number of (transport) equations solved for to compute the eddy viscosity coefficient.

 model
model
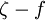 model
model
![- \rho \left\langle u_{i} u_{j} \right\rangle = \mu_{t} \left[ S_{ij} - \frac{1}{3} S_{kk} \delta_{ij} \right]](/W/images/math/5/f/8/5f8568434101e54fd4cb409ab338744a.png)
![S_{ij}= \frac{1}{2} \left[ \frac{\partial U_{i}}{\partial x_{j}} + \frac{\partial U_{j}}{\partial x_{i}} \right]](/W/images/math/1/b/a/1baa28f3f8103315b8311820780a8205.png)