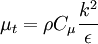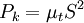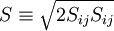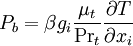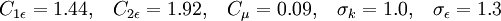CFD Online Logo CFD Online URL
www.cfd-online.com
[Sponsors]
Home > Wiki > Standard k-epsilon model

Standard k-epsilon model

From CFD-Wiki

(Difference between revisions)
Jump to: navigation, search
(Someone added that C3 was -0.33, without any reference. This can be confusing to OpenFOAM users, because their k-epsilon has another C3, for another reason.)
 
(16 intermediate revisions not shown)
Line 1: Line 1:
-
== Transport Equations for standard k-epsilon model ==
+
{{Turbulence modeling}}
-
For k <br>
+
== Transport equations for standard k-epsilon model ==
-
<math>  \frac{\partial}{\partial t} (\rho k) + \frac{\partial}{\partial x_i} (\rho k u_i) = \frac{\partial}{\partial x_j} \left[ \left(\mu + \frac{\mu_t}{\sigma_k} \right) \frac{\partial k}{\partial x_j}\right] + P_k + P_b - \rho \epsilon - Y_M + S_k  </math>
+
 
 +
For turbulent kinetic energy <math> k </math> <br>
 +
:<math>  \frac{\partial}{\partial t} (\rho k) + \frac{\partial}{\partial x_i} (\rho k u_i) = \frac{\partial}{\partial x_j} \left[ \left(\mu + \frac{\mu_t}{\sigma_k} \right) \frac{\partial k}{\partial x_j}\right] + P_k + P_b - \rho \epsilon - Y_M + S_k  </math>
<br>
<br>
Line 8: Line 10:
<br>
<br>
-
<math>  
+
:<math>  
\frac{\partial}{\partial t} (\rho \epsilon) + \frac{\partial}{\partial x_i} (\rho \epsilon u_i) = \frac{\partial}{\partial x_j} \left[\left(\mu + \frac{\mu_t}{\sigma_{\epsilon}} \right) \frac{\partial \epsilon}{\partial x_j} \right] + C_{1 \epsilon}\frac{\epsilon}{k} \left( P_k + C_{3 \epsilon} P_b \right) - C_{2 \epsilon} \rho \frac{\epsilon^2}{k} + S_{\epsilon}
\frac{\partial}{\partial t} (\rho \epsilon) + \frac{\partial}{\partial x_i} (\rho \epsilon u_i) = \frac{\partial}{\partial x_j} \left[\left(\mu + \frac{\mu_t}{\sigma_{\epsilon}} \right) \frac{\partial \epsilon}{\partial x_j} \right] + C_{1 \epsilon}\frac{\epsilon}{k} \left( P_k + C_{3 \epsilon} P_b \right) - C_{2 \epsilon} \rho \frac{\epsilon^2}{k} + S_{\epsilon}
-
 
+
</math>
-
</math>
+
== Modeling turbulent viscosity ==
== Modeling turbulent viscosity ==
Turbulent viscosity is modelled as: <br>
Turbulent viscosity is modelled as: <br>
-
<math>
+
:<math>
\mu_t = \rho C_{\mu} \frac{k^2}{\epsilon}
\mu_t = \rho C_{\mu} \frac{k^2}{\epsilon}
</math>
</math>
-
<br>
 
-
 
-
 
-
 
== Production of k ==
== Production of k ==
-
<math>
+
:<math>
P_k = - \rho \overline{u'_i u'_j} \frac{\partial u_j}{\partial x_i}   
P_k = - \rho \overline{u'_i u'_j} \frac{\partial u_j}{\partial x_i}   
</math>
</math>
<br>
<br>
-
<math> P_k = \mu_t S^2 </math>
+
:<math> P_k = \mu_t S^2 </math>
Where <math> S </math> is the modulus of the mean rate-of-strain tensor, defined as : <br>
Where <math> S </math> is the modulus of the mean rate-of-strain tensor, defined as : <br>
-
<math>
+
:<math>
S \equiv \sqrt{2S_{ij} S_{ij}}  
S \equiv \sqrt{2S_{ij} S_{ij}}  
</math>
</math>
-
== Effect of Bouyancy ==
+
== Effect of buoyancy ==
-
<math>
+
:<math>
P_b = \beta g_i \frac{\mu_t}{{\rm Pr}_t} \frac{\partial T}{\partial x_i}  
P_b = \beta g_i \frac{\mu_t}{{\rm Pr}_t} \frac{\partial T}{\partial x_i}  
</math>
</math>
Line 47: Line 44:
The coefficient of thermal expansion, <math> \beta </math> , is defined as <br>
The coefficient of thermal expansion, <math> \beta </math> , is defined as <br>
-
<math>  
+
:<math>  
\beta = - \frac{1}{\rho} \left(\frac{\partial \rho}{\partial T}\right)_p  
\beta = - \frac{1}{\rho} \left(\frac{\partial \rho}{\partial T}\right)_p  
</math>
</math>
-
== Model Constants ==
+
== Model constants ==
-
<math>
+
:<math>
-
C_{1 \epsilon} = 1.44, \;\; C_{2 \epsilon} = 1.92, \;\; C_{\mu} = 0.09, \;\; \sigma_k = 1.0, \;\; \sigma_{\epsilon} = 1.3  
+
C_{1 \epsilon} = 1.44, \;\;\; C_{2 \epsilon} = 1.92,\;\; \; C_{\mu} = 0.09, \;\;\; \sigma_k = 1.0, \;\;\; \sigma_{\epsilon} = 1.3  
</math>
</math>
 +
 +
 +
'''Note''': <math>C_{3 \epsilon}</math> depends on the literature being followed and is meant to be used only with the <math>P_b</math> term. Possible values, depending on literature reference:
 +
 +
{| class="wikitable"
 +
|- align="center"
 +
! Reference !! Constant !! Comments
 +
|- align="center"
 +
| ''unknown'' || <math>C_{3 \epsilon} = -0.33</math> || Note to OpenFOAM users: do not confuse this constant with the one used in their implementations of the k-epsilon turbulence models. Their implementation is different.
 +
|}
 +
 +
 +
== References ==
 +
 +
See section [[K-epsilon_models#References|References]] in the parent page [[K-epsilon models]].
 +
 +
 +
[[Category:Turbulence models]]

Latest revision as of 20:15, 16 December 2014

Turbulence modeling
Turbulence
RANS-based turbulence models
  1. Linear eddy viscosity models
    1. Algebraic models
      1. Cebeci-Smith model
      2. Baldwin-Lomax model
      3. Johnson-King model
      4. A roughness-dependent model
    2. One equation models
      1. Prandtl's one-equation model
      2. Baldwin-Barth model
      3. Spalart-Allmaras model
    3. Two equation models
      1. k-epsilon models
        1. Standard k-epsilon model
        2. Realisable k-epsilon model
        3. RNG k-epsilon model
        4. Near-wall treatment
      2. k-omega models
        1. Wilcox's k-omega model
        2. Wilcox's modified k-omega model
        3. SST k-omega model
        4. Near-wall treatment
      3. Realisability issues
        1. Kato-Launder modification
        2. Durbin's realizability constraint
        3. Yap correction
        4. Realisability and Schwarz' inequality
  2. Nonlinear eddy viscosity models
    1. Explicit nonlinear constitutive relation
      1. Cubic k-epsilon
      2. EARSM
    2. v2-f models
      1. \overline{\upsilon^2}-f model
      2. \zeta-f model
  3. Reynolds stress model (RSM)
Large eddy simulation (LES)
  1. Smagorinsky-Lilly model
  2. Dynamic subgrid-scale model
  3. RNG-LES model
  4. Wall-adapting local eddy-viscosity (WALE) model
  5. Kinetic energy subgrid-scale model
  6. Near-wall treatment for LES models
Detached eddy simulation (DES)
Direct numerical simulation (DNS)
Turbulence near-wall modeling
Turbulence free-stream boundary conditions
  1. Turbulence intensity
  2. Turbulence length scale

Contents

Transport equations for standard k-epsilon model

For turbulent kinetic energy k

\frac{\partial}{\partial t} (\rho k) + \frac{\partial}{\partial x_i} (\rho k u_i) = \frac{\partial}{\partial x_j} \left[ \left(\mu + \frac{\mu_t}{\sigma_k} \right) \frac{\partial k}{\partial x_j}\right] + P_k + P_b - \rho \epsilon - Y_M + S_k


For dissipation \epsilon

\frac{\partial}{\partial t} (\rho \epsilon) + \frac{\partial}{\partial x_i} (\rho \epsilon u_i) = \frac{\partial}{\partial x_j} \left[\left(\mu + \frac{\mu_t}{\sigma_{\epsilon}} \right) \frac{\partial \epsilon}{\partial x_j} \right] + C_{1 \epsilon}\frac{\epsilon}{k} \left( P_k + C_{3 \epsilon} P_b \right) - C_{2 \epsilon} \rho \frac{\epsilon^2}{k} + S_{\epsilon}

Modeling turbulent viscosity

Turbulent viscosity is modelled as:

\mu_t = \rho C_{\mu} \frac{k^2}{\epsilon}

Production of k

P_k = - \rho \overline{u'_i u'_j} \frac{\partial u_j}{\partial x_i}


P_k = \mu_t S^2

Where S is the modulus of the mean rate-of-strain tensor, defined as :

S \equiv \sqrt{2S_{ij} S_{ij}}

Effect of buoyancy

P_b = \beta g_i \frac{\mu_t}{{\rm Pr}_t} \frac{\partial T}{\partial x_i}



where Prt is the turbulent Prandtl number for energy and gi is the component of the gravitational vector in the ith direction. For the standard and realizable - models, the default value of Prt is 0.85.

The coefficient of thermal expansion, \beta , is defined as

\beta = - \frac{1}{\rho} \left(\frac{\partial \rho}{\partial T}\right)_p

Model constants

C_{1 \epsilon} = 1.44, \;\;\; C_{2 \epsilon} = 1.92,\;\; \; C_{\mu} = 0.09, \;\;\; \sigma_k = 1.0, \;\;\; \sigma_{\epsilon} = 1.3


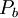Note: C_{3 \epsilon} depends on the literature being followed and is meant to be used only with the P_b term. Possible values, depending on literature reference:

Reference Constant Comments
unknown 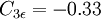C_{3 \epsilon} = -0.33 Note to OpenFOAM users: do not confuse this constant with the one used in their implementations of the k-epsilon turbulence models. Their implementation is different.


References

See section References in the parent page K-epsilon models.

Retrieved from "https://openfoam.cfd-online.com/Wiki/Standard_k-epsilon_model"
My wiki