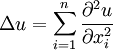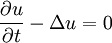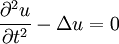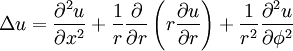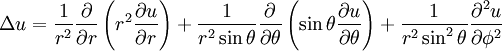Laplacian

From CFD-Wiki

Revision as of 08:19, 12 April 2007 by Jola (Talk | contribs)

(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)

The n-dimensional Laplacian operator in Cartesian coordinates is defined by

$\Delta u = \sum_{i=1}^n \frac{\partial^2 u}{\partial x_i^2}$

It is an important differential operator which occurs in many equations of mathematical physics and is usually associated with dissipative effects (except in the case of wave equation). Some of the important equations are

Laplace equation

$\Delta u = 0$

Poisson equation

$-\Delta u = f$

Heat equation

$\frac{\partial u}{\partial t} - \Delta u = 0$

Wave equation

$\frac{\partial^2 u}{\partial t^2} - \Delta u = 0$

Solutions of these equations are very smooth and in most cases are infinitely differentiable (when the associated data of the problem are sufficiently smooth).

Folland (see reference below) explains the ubiquitous appearance of the Laplacian.

Why is it so ubiquitous ? The answer, which we shall prove, is that it commutes with translations and rotations and generates the ring of all differential operators with this property. Hence, the Laplacian is likely to turn up in the description of any physical process whose underlying physics is homogeneous (independent of position) and isotropic (independent of direction).

Moreover it can shown that any linear operator which commutes with translations and rotations must be a polynomial in $\Delta$ , i.e., it must be of the form $\sum_j a_j \Delta^j$ where the $a_j$ are constants (see Folland).

The Laplacian operator is invariant under coordinate translation and rotation.

The Laplace operator is also denoted as $\nabla^2$ since it is the divergence of the gradient operator

$\Delta = \nabla^2 = \nabla \cdot \nabla$

Laplacian in cylindrical coordinates

If 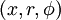$(x,r,\phi)$ are cylindrical coordinates, then the Laplacian of a scalar field variable $u$ is

$\Delta u = \frac{\partial^2 u}{\partial x^2} + \frac{1}{r} \frac{\partial}{\partial r} \left( r \frac{\partial u}{\partial r} \right) + \frac{1}{r^2} \frac{\partial^2 u}{\partial \phi^2}$

Laplacian in spherical coordinates

If 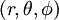$(r,\theta,\phi)$ are spherical coordinates, then the Laplacian of a scalar field variable $u$ is

$\Delta u = \frac{1}{r^2} \frac{\partial}{\partial r} \left( r^2 \frac{\partial u}{\partial r} \right) + \frac{1}{r^2 \sin\theta} \frac{\partial}{\partial \theta} \left( \sin\theta \frac{\partial u}{\partial \theta} \right) + \frac{1}{r^2 \sin^2\theta} \frac{\partial^2 u}{\partial \phi^2}$

References

Gerald B. Folland (1995), Introduction to partial differential equations, Princeton University Press.

Laplacian

From CFD-Wiki

Laplacian in cylindrical coordinates

Laplacian in spherical coordinates

References

Views

My wiki

wiki navigation

wiki search

wiki toolbox