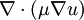Generic scalar transport equation

From CFD-Wiki

A differential equation epxresses a certain conservation principle. Whether be it in electromagnetics, fluid dynamics, heat transfer, radiation, electronics... conservation principles are the basis for the derivation of differential or integro-differential equations. In this respect, any differential equation addresses a certain quantity as it dependent variable and thus expresses the balance between the phenomena affecting the evolution of this quanitity. For example, the temperature of a fluid in a heated pipe is affected by convection due to the solid-fluid interface, and due to the fluid-fluid interaction. Furthermore, temperature is also diffused inside the fluid. For a state state problem, with the absence of sources, a differential equation governing the temperature will definetely express a balance between convection and diffusion.
A brief inspection of the equations governing various physical phenomena will reveal that all of these equations can be put into a generic form thus allowing a systematic approach for a computer simulation.
For example, the conservation equation of a chemical species 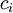is
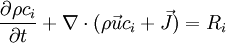where 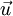denotes the velocity field, 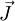denotes the diffusion flux the of the chemical species, and 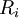denotes the rate of generation of 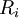caused by the chemical reaction.
The x-momentum equation for a Newtonian fluid can be written as

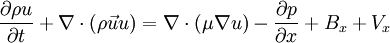
where is the body force in the x-direction and 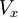includes the viscous terms that are not expressed by