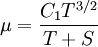Sutherland's law
From CFD-Wiki
(Difference between revisions)
| Line 1: | Line 1: | ||
| - | In 1893 [http://en.wikipedia.org/wiki/William_Sutherland_(physicist) William Sutherland], an Australian physicist, published a relationship between the | + | In 1893 [http://en.wikipedia.org/wiki/William_Sutherland_(physicist) William Sutherland], an Australian physicist, published a relationship between the dynamic visocity, <math>\mu</math>, and the absolute temperature, <math>T</math>, of an ideal gas. This formula, often called Sutherland's law, is based on kinetic theory of ideal gases and an idealized intermolecular-force potential. Sutherland's law is still commonly used and most often gives fairly accurate results with an error less than a few percent over a wide range of temperatures. Sutherland's law can be expressed as: |
:<math>\mu = \mu_r \left( \frac{T}{T_r} \right)^{3/2}\frac{T_r + S}{T + S}</math> | :<math>\mu = \mu_r \left( \frac{T}{T_r} \right)^{3/2}\frac{T_r + S}{T + S}</math> | ||
| Line 14: | Line 14: | ||
:<math>C_1 = \frac{\mu_r}{T_r^{3/2}}(T_r + S)</math> | :<math>C_1 = \frac{\mu_r}{T_r^{3/2}}(T_r + S)</math> | ||
| + | |||
| + | {| align=center border=1 | ||
| + | |+ Sutherland's law coefficients | ||
| + | ! Gas !! <math>\mu_0 [\frac{kg}{m s}]</math> !! <math>T_0 [K]</math> !! <math>S [K]</math> !! <math>C_1 [\frac{kg}{m s k ^ {0.5}}]</math> | ||
| + | |- | ||
| + | | Air | ||
| + | | <math>17.16*10^{-6}</math> | ||
| + | | 0 | ||
| + | | 0 | ||
| + | | 0 | ||
| + | |} | ||
== References == | == References == | ||
* {{reference-paper|author=Sutherland, W.|year=1893|title=The viscosity of gases and molecular force|rest=Philosophical Magazine, S. 5, 36, pp. 507-531 (1893)}} | * {{reference-paper|author=Sutherland, W.|year=1893|title=The viscosity of gases and molecular force|rest=Philosophical Magazine, S. 5, 36, pp. 507-531 (1893)}} | ||
Revision as of 14:16, 17 May 2007
In 1893 William Sutherland, an Australian physicist, published a relationship between the dynamic visocity, 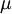 , and the absolute temperature,
, and the absolute temperature, 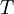 , of an ideal gas. This formula, often called Sutherland's law, is based on kinetic theory of ideal gases and an idealized intermolecular-force potential. Sutherland's law is still commonly used and most often gives fairly accurate results with an error less than a few percent over a wide range of temperatures. Sutherland's law can be expressed as:
, of an ideal gas. This formula, often called Sutherland's law, is based on kinetic theory of ideal gases and an idealized intermolecular-force potential. Sutherland's law is still commonly used and most often gives fairly accurate results with an error less than a few percent over a wide range of temperatures. Sutherland's law can be expressed as:
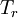 is a reference temperature.
is a reference temperature.
 is the viscosity at the
is the viscosity at the 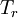 reference temperature
reference temperature
- S is the Sutherland temperature
Some authors instead express Sutherland's law in the following form:
Comparing the formulas above the 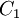 constant can be written as:
constant can be written as:
| Gas | ![\mu_0 [\frac{kg}{m s}]](/W/images/math/2/4/8/2480f45fd1c786230d032f54d5a4611f.png) | ![T_0 [K]](/W/images/math/1/e/6/1e63651a7e3249da3521fb3d1523c542.png) | ![S [K]](/W/images/math/e/4/4/e444c70ca8ff28fe00474e6ee27b3872.png) | ![C_1 [\frac{kg}{m s k ^ {0.5}}]](/W/images/math/a/f/a/afaee00bd73585024408c1f5b591b06e.png)
|
|---|---|---|---|---|
| Air | 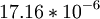
| 0 | 0 | 0 |
References
- Sutherland, W. (1893), "The viscosity of gases and molecular force", Philosophical Magazine, S. 5, 36, pp. 507-531 (1893).