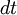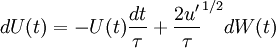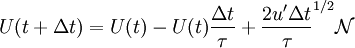Langevin equation
From CFD-Wiki
(Difference between revisions)
m |
|||
| Line 18: | Line 18: | ||
The Wiener process can be understood as Gaussian random variable with 0 mean | The Wiener process can be understood as Gaussian random variable with 0 mean | ||
and variance <math> dt</math> | and variance <math> dt</math> | ||
| + | |||
| + | {{Stub}} | ||
Revision as of 09:29, 14 November 2005
The stochastic differential equation (SDE) for velocity component 
,
the Langevin equation is
where 
is a Wiener process.
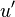is the turbulence intensity and 
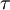a Lagrangian time-scale.
Th finite difference approximation of the above equation is
where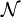 
is a standardized Gaussian random variable with 0 mean an unity variance
which is independent of 
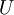on all other time steps (Pope 1994).
The Wiener process can be understood as Gaussian random variable with 0 mean
and variance 
