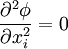Potential flow
From CFD-Wiki
(Difference between revisions)
m (→External Links: Fixed broken link) |
(Gouverning equations modified) |
||
| Line 15: | Line 15: | ||
== Governing equations == | == Governing equations == | ||
| + | In the case of an incompressible flow, <math>\phi</math> satisfies the Laplace equation. | ||
| + | From the continuity equation, we get: | ||
| + | :<math> | ||
| + | \frac{\partial^2 \phi}{\partial x_i^2}=0 | ||
| + | </math> | ||
== External Links == | == External Links == | ||
* [http://simscience.org/fluid/red/superpos.html Applet Simulating 2D Potential Flow] | * [http://simscience.org/fluid/red/superpos.html Applet Simulating 2D Potential Flow] | ||
Revision as of 08:20, 20 August 2013
A flow in which vorticity is zero is called potential flow, or irrotational flow. Since the vorticity is zero
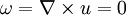
it implies that the velocity is the gradient of a scalar field called the velocity potential, and usually denoted as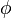 
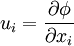
At high Reynolds numbers, flow past slender bodies is attached (no boundary layer separation) and the boundary layers are thin. In such situations vorticity is confined to the thin boundary layers and the rest of the flow is irrotational.
Governing equations
In the case of an incompressible flow, 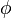satisfies the Laplace equation.
From the continuity equation, we get:
