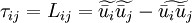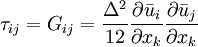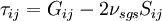Structural modeling
From CFD-Wiki
(Difference between revisions)
| Line 2: | Line 2: | ||
:<math> | :<math> | ||
| - | \tau_{ij} = \widetilde{\bar{u}_i} \widetilde{\bar{u}_j} - \widetilde{\bar{u}_i \bar{u}_j} | + | \tau_{ij} = L_{ij} = \widetilde{\bar{u}_i} \widetilde{\bar{u}_j} - \widetilde{\bar{u}_i \bar{u}_j} |
</math> | </math> | ||
| Line 9: | Line 9: | ||
:<math> | :<math> | ||
| - | \tau_{ij} = \frac{\Delta^2}{12} \frac{\partial \bar{u}_i}{\partial x_{k}} \frac{\partial \bar{u}_j}{\partial x_{k}} | + | \tau_{ij} = G_{ij} = \frac{\Delta^2}{12} \frac{\partial \bar{u}_i}{\partial x_{k}} \frac{\partial \bar{u}_j}{\partial x_{k}} |
| + | </math> | ||
| + | |||
| + | Mixed models, which are based on linear combinations of the eddy-viscosity and structural types | ||
| + | |||
| + | :<math> | ||
| + | \tau_{ij} = G_{ij}-2\nu_{sgs} S_{ij} | ||
| + | </math> | ||
| + | or | ||
| + | :<math> | ||
| + | \tau_{ij} = L_{ij}-2\nu_{sgs} S_{ij} | ||
</math> | </math> | ||
Revision as of 22:06, 24 June 2013
Those that use the physical hypothesis of scale similarity
Those derived by formal series expansions
Mixed models, which are based on linear combinations of the eddy-viscosity and structural types
or