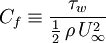Skin friction coefficient
From CFD-Wiki
(Difference between revisions)
| Line 5: | Line 5: | ||
Where <math>\tau_w</math> is the local [[wall shear stress]], <math>\rho</math> is the fluid density and <math>U_\infty</math> is the free-stream velocity (usually taken ouside of the boundary layer or at the inlet). | Where <math>\tau_w</math> is the local [[wall shear stress]], <math>\rho</math> is the fluid density and <math>U_\infty</math> is the free-stream velocity (usually taken ouside of the boundary layer or at the inlet). | ||
| - | For a turbulent boundary layer several approximation formulas for the local skin friction can be used: | + | For a turbulent boundary layer several approximation formulas for the local skin friction for a flat plate can be used: |
1/7 power law: | 1/7 power law: | ||
| Line 17: | Line 17: | ||
Schlichting (equation 21.16 footnote in [1]) | Schlichting (equation 21.16 footnote in [1]) | ||
| - | <math>C_f = [ 2 \, | + | <math>C_f = [ 2 \, log_{10}(Re_x) - 0.65 ] ^{-2.3} \quad \mbox{for} \quad Re_x < 10^9 </math> |
Schultz-Grunov (equation 21.19a in [1]): | Schultz-Grunov (equation 21.19a in [1]): | ||
| - | <math>C_f = 0.370 \, [ | + | <math>C_f = 0.370 \, [ log_{10}(Re_x) ]^{-2.584} </math> |
| + | |||
| + | (equation 38 in [2]): | ||
| + | |||
| + | <math>1.0/C_f^{1/2} = 1.7 + 4.15 \, log_{10} (Re_x \, C_f) </math> | ||
== References == | == References == | ||
# {{reference-book|author=Schlichting, Hermann |year=1979|title=Boundary Layer Theory|rest=ISBN 0-07-055334-3, 7th Edition}} | # {{reference-book|author=Schlichting, Hermann |year=1979|title=Boundary Layer Theory|rest=ISBN 0-07-055334-3, 7th Edition}} | ||
| + | # {{reference-book|author=von Karman, Theodore |year=1934|title=Turbulence and Skin Friction|rest=J. of the Aeronautical Sciences, Vol. 1, No 1, 1934, pp. 1-20}} | ||
== To do == | == To do == | ||
Revision as of 17:12, 28 February 2011
The skin friction coefficient,  , is defined by:
, is defined by:
Where 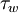 is the local wall shear stress,
is the local wall shear stress, 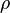 is the fluid density and
is the fluid density and  is the free-stream velocity (usually taken ouside of the boundary layer or at the inlet).
is the free-stream velocity (usually taken ouside of the boundary layer or at the inlet).
For a turbulent boundary layer several approximation formulas for the local skin friction for a flat plate can be used:
1/7 power law:
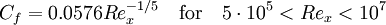
1/7 power law with experimental calibration (equation 21.12 in [1]):
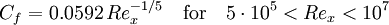
Schlichting (equation 21.16 footnote in [1])
![C_f = [ 2 \, log_{10}(Re_x) - 0.65 ] ^{-2.3} \quad \mbox{for} \quad Re_x < 10^9](/W/images/math/c/a/4/ca4dc895c55260e9f9244eb0465141c8.png)
Schultz-Grunov (equation 21.19a in [1]):
![C_f = 0.370 \, [ log_{10}(Re_x) ]^{-2.584}](/W/images/math/3/4/4/34492727370cbb6fa12a571f65a1388a.png)
(equation 38 in [2]):
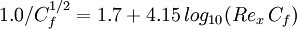
References
- Schlichting, Hermann (1979), Boundary Layer Theory, ISBN 0-07-055334-3, 7th Edition.
- von Karman, Theodore (1934), Turbulence and Skin Friction, J. of the Aeronautical Sciences, Vol. 1, No 1, 1934, pp. 1-20.
To do
Someone should add more data about total skin friction approximations, Prandtl-Schlichting skin-friction formula, and the Karman-Schoenherr equation.