Kato-Launder modification
From CFD-Wiki
m |
|||
| Line 19: | Line 19: | ||
P = \tau_{ij}^{turb} \frac{\partial u_i}{\partial x_j} | P = \tau_{ij}^{turb} \frac{\partial u_i}{\partial x_j} | ||
</math> | </math> | ||
| - | Where <math>\tau_{ij}^{turb}</math> is the turbulent shear stress tensor</math> | + | Where <math>\tau_{ij}^{turb}</math> is the turbulent shear stress tensor given by the Boussinesq assumption: |
| + | |||
| + | :<math> | ||
| + | \tau_{ij}^{turb} \defeq | ||
| + | - \overline{\rho u''_i u''_j} \approx | ||
| + | 2 \mu_t \widetilde{S_{ij}^*} - | ||
| + | \frac{2}{3} \rav{\rho} k \delta_{ij} | ||
| + | </math> | ||
| + | |||
| + | |||
| + | |||
==References== | ==References== | ||
{{reference-paper|author=Kato, M. and Launder, B. E.|year=1993|title=The Modeling of Turbulent Flow Around Stationary and Vibrating Square Cylinders|rest=Proc. 9th Symposium on Turbulent Shear Flows, Kyoto, August 1993, pp. 10.4.1-10.4.6}} | {{reference-paper|author=Kato, M. and Launder, B. E.|year=1993|title=The Modeling of Turbulent Flow Around Stationary and Vibrating Square Cylinders|rest=Proc. 9th Symposium on Turbulent Shear Flows, Kyoto, August 1993, pp. 10.4.1-10.4.6}} | ||
Revision as of 14:38, 8 December 2005
The Kato-Launder modification is an ad-hoc modification of the turbulent production term in the k equation. The main purpose of the modification is to reduce the tendency that two-equation models have to over-predict the turbulent production in regions with large normal strain, i.e. regions with strong acceleration or decelleration.
The transport equation for the turbulent energy, 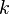 , used in most two-equation models can be written as:
, used in most two-equation models can be written as:
Where  is the turbulent production normally given by:
is the turbulent production normally given by:
Where 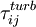 is the turbulent shear stress tensor given by the Boussinesq assumption:
is the turbulent shear stress tensor given by the Boussinesq assumption:
- Failed to parse (unknown function\defeq): \tau_{ij}^{turb} \defeq - \overline{\rho u''_i u''_j} \approx 2 \mu_t \widetilde{S_{ij}^*} - \frac{2}{3} \rav{\rho} k \delta_{ij}
References
Kato, M. and Launder, B. E. (1993), "The Modeling of Turbulent Flow Around Stationary and Vibrating Square Cylinders", Proc. 9th Symposium on Turbulent Shear Flows, Kyoto, August 1993, pp. 10.4.1-10.4.6.

![\frac{\partial}{\partial t} \left( \rho k \right) +
\frac{\partial}{\partial x_j}
\left[
\rho k u_j - \left( \mu + \frac{\mu_t}{\sigma_k} \right)
\frac{\partial k}{\partial x_j}
\right]
=
P - \rho \epsilon - \rho D](/W/images/math/8/d/7/8d787db6600e44ff6855992620431595.png)
