Wall-adapting local eddy-viscosity (WALE) model

(Difference between revisions)

Latest revision as of 08:31, 19 May 2018

In the WALE model the eddy viscosity is modeled by:

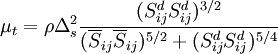
$\mu_{t} = \rho \Delta _s^2 \frac{(S_{ij}^{d} S_{ij}^{d})^{3/2}}{(\overline{S}_{ij} \overline{S}_{ij})^{5/2} + (S_{ij}^{d} S_{ij}^{d})^{5/4}}$

$\Delta _s = C_w V^{1/3}$

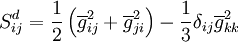
$S_{ij}^{d} = \frac{1}{2} \left( \overline{g}_{ij}^{2} + \overline{g}_{ji}^{2} \right) - \frac{1}{3} \delta_{ij} \overline{g}_{kk}^{2}$

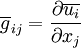
$\overline{g}_{ij} = \frac{\partial \overline{u_i}}{\partial x_{j}}$

$\overline{g}_{ij}^{2} = \overline{g}_{ik} \overline{g}_{kj}$

where 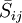$\bar S_{ij}$ is the rate-of-strain tensor for the resolved scale defined by

$\bar S_{ij} = \frac{1}{2}\left( {\frac{{\partial \bar u_i }}{{\partial x_j }} + \frac{{\partial \bar u_j }}{{\partial x_i }}} \right)$

Where the constant $C_w = 0.325$

@@ Line 9: / Line 9: @@
 <math> S_{ij}^{d} = \frac{1}{2} \left( \overline{g}_{ij}^{2} + \overline{g}_{ji}^{2}  \right) - \frac{1}{3} \delta_{ij} \overline{g}_{kk}^{2}  </math>
-<math>  \overline{g}_{ij} = \frac{\partial \overline{u_i}}{\partial x_{j}}, </math>
+<math>  \overline{g}_{ij} = \frac{\partial \overline{u_i}}{\partial x_{j}} </math>
 <math>  \overline{g}_{ij}^{2} = \overline{g}_{ik} \overline{g}_{kj}        </math>