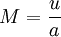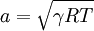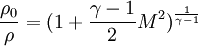Isentropic flow relations
From CFD-Wiki
(Difference between revisions)
m (Isentropic Flow Relations moved to Isentropic flow relations) |
|||
| Line 1: | Line 1: | ||
| - | <math>M = \frac{ | + | ''Below are the formulas... someone needs to add some explaining text here'' |
| + | |||
| + | <math>M = \frac{u}{a}</math> | ||
<math>a = \sqrt{\gamma R T}</math> | <math>a = \sqrt{\gamma R T}</math> | ||
Revision as of 12:30, 13 September 2005
Below are the formulas... someone needs to add some explaining text here