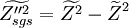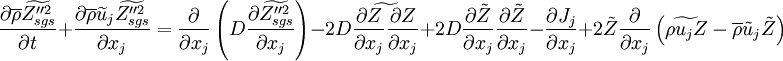Subgrid variance
From CFD-Wiki
(Difference between revisions)
m |
|||
| Line 7: | Line 7: | ||
An equation for the subgrid variance is | An equation for the subgrid variance is | ||
| + | :<math> | ||
| + | \frac{\partial \overline{\rho} \widetilde{Z_{sgs}''^2} }{\partial t} + | ||
| + | \frac{\partial \overline{\rho} \widetilde{u}_j \widetilde{Z_{sgs}''^2} }{\partial x_j}= | ||
| + | \frac{\partial}{\partial x_j}\left(D \frac{\partial \widetilde{Z_{sgs}''^2} }{\partial x_j} \right) | ||
| + | -2 D \widetilde{\frac{\partial Z}{\partial x_j}\frac{\partial Z }{\partial x_j}} | ||
| + | + 2 D \frac{\partial \tilde{Z}}{\partial x_j}\frac{\partial \tilde{Z}}{\partial x_j} - | ||
| + | \frac{\partial J_j}{\partial x_j} + | ||
| + | 2 \tilde{Z} \frac{\partial}{\partial x_j} | ||
| + | \left( \widetilde{\rho u_j Z}- \overline{\rho}\tilde{u}_j \tilde{Z} \right) | ||
| + | </math> | ||
| + | |||
| + | where <math> J_j = \widetilde{\rho u_j {Z_{sgs}''^2}}- \overline{\rho} \tilde{u_j} \widetilde{Z_{sgs}''^2} </math> is a subgrid variance flux and is often modeled using a gradient approach with turbulent diffusivity. | ||
Instead of solving the above equation, algebraic models are often used. | Instead of solving the above equation, algebraic models are often used. | ||
Latest revision as of 11:19, 11 January 2006
The subgrid variance of a passive scalar is defined as
The scalar subgrid variance is also known as the subgrid scalar energy in analogy to the kinetic subgrid energy. An equation for the subgrid variance is
where 
is a subgrid variance flux and is often modeled using a gradient approach with turbulent diffusivity.
Instead of solving the above equation, algebraic models are often used. For dimensional analysis
where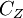 
can be obtained from the scalar spectra and its value is 0.1-0.2.
