Introduction to turbulence/Statistical analysis/Multivariate random variables
From CFD-Wiki
(Difference between revisions)
(→Joint pdfs and joint moments) |
|||
| Line 2: | Line 2: | ||
Often it is importamt to consider more than one random variable at a time. For example, in turbulence the three components of the velocity vector are interralated and must be considered together. In addition to the ''marginal'' (or single variable) statistical moments already considered, it is necessary to consider the '''joint''' statistical moments. | Often it is importamt to consider more than one random variable at a time. For example, in turbulence the three components of the velocity vector are interralated and must be considered together. In addition to the ''marginal'' (or single variable) statistical moments already considered, it is necessary to consider the '''joint''' statistical moments. | ||
| + | |||
| + | For example if <math>u</math> and <math>v</math> are two random variables, there are three second-order moments which can be defined <math>\left\langle u^{2} \right\rangle </math> , <math>\left\langle v^{2} \right\rangle </math> , and <math>\left\langle uv \right\rangle </math>. The product moment <math>\left\langle uv \right\rangle </math> is called the ''cross-correlation'' or ''cross-covariance''. The moments | ||
=== The bi-variate normal (or Gaussian) distribution === | === The bi-variate normal (or Gaussian) distribution === | ||
dssd | dssd | ||
Revision as of 18:21, 1 June 2006
Joint pdfs and joint moments
Often it is importamt to consider more than one random variable at a time. For example, in turbulence the three components of the velocity vector are interralated and must be considered together. In addition to the marginal (or single variable) statistical moments already considered, it is necessary to consider the joint statistical moments.
For example if 
and 
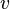are two random variables, there are three second-order moments which can be defined 
, 
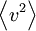, and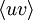 
. The product moment 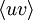is called the cross-correlation or cross-covariance. The moments
The bi-variate normal (or Gaussian) distribution
dssd
