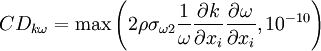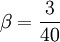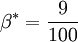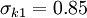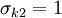SST k-omega model
From CFD-Wiki
(Difference between revisions)
(→Closure Coefficients and Auxilary Relations) |
(→Closure Coefficients and Auxilary Relations) |
||
| Line 17: | Line 17: | ||
:<math> | :<math> | ||
| - | + | F_2=\mbox{tanh} \left[ \left[ \mbox{max} \left( { 2 \sqrt{k} \over \beta^* \omega y } , { 500 \nu \over y^2 \omega } \right) \right]^2 \right] | |
</math> | </math> | ||
:<math> | :<math> | ||
| - | + | P_k=\mbox{min} \left(\tau _{ij} {{\partial U_i } \over {\partial x_j }} , 20\beta^* k \omega \right) | |
</math> | </math> | ||
:<math> | :<math> | ||
| - | + | F_1=\mbox{tanh} \left\{ \left\{ \mbox{min} \left[ \mbox{max} \left( {\sqrt{k} \over \beta ^* \omega y}, {500 \nu \over y^2 \omega} \right) , {4 \sigma_{\omega 2} k \over CD_{k\omega} y^2} \right] \right\} ^4 \right\} | |
</math> | </math> | ||
| - | |||
:<math> | :<math> | ||
| - | + | CD_{k\omega}=\mbox{max} \left( 2\rho\sigma_{\omega 2} {1 \over \omega} {{\partial k} \over {\partial x_i}} {{\partial \omega} \over {\partial x_i}}, 10 ^{-10} \right ) | |
</math> | </math> | ||
| Line 47: | Line 46: | ||
:<math> | :<math> | ||
| - | \ | + | \sigma_{k1} = 0.85 |
| + | </math> | ||
| + | |||
| + | :<math> | ||
| + | \sigma_{k2} = 1 | ||
</math> | </math> | ||
:<math> | :<math> | ||
| - | \ | + | \sigma_{\omega 1} = 0.5 |
</math> | </math> | ||
:<math> | :<math> | ||
| - | \ | + | \sigma_{\omega 2} = 0.856 |
</math> | </math> | ||
Revision as of 08:11, 11 October 2005
Contents |
Kinematic Eddy Viscosity
Turbulence Kinetic Energy
Specific Dissipation Rate
Closure Coefficients and Auxilary Relations
References
- Wilcox, D.C. (1988), "Re-assessment of the scale-determining equation for advanced turbulence models", AIAA Journal, vol. 31, pp. 1414-1421.

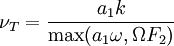
![{{\partial k} \over {\partial t}} + U_j {{\partial k} \over {\partial x_j }} = P_k - \beta ^* k\omega + {\partial \over {\partial x_j }}\left[ {\left( {\nu + \sigma_{k1} \nu _T } \right){{\partial k} \over {\partial x_j }}} \right]](/W/images/math/2/6/0/260b982db012f9ae1575154ade40b566.png)
![{{\partial \omega } \over {\partial t}} + U_j {{\partial \omega } \over {\partial x_j }} = \alpha S^2 - \beta \omega ^2 + {\partial \over {\partial x_j }}\left[ {\left( {\nu + \sigma_{\omega 1} \nu _T } \right){{\partial \omega } \over {\partial x_j }}} \right] + 2( 1 - F_1 ) \sigma_{\omega 2} {1 \over \omega} {{\partial k } \over {\partial x_i}} {{\partial \omega } \over {\partial x_i}}](/W/images/math/1/d/7/1d76c55d743546e29debcc8945e5d4e6.png)
![F_2=\mbox{tanh} \left[ \left[ \mbox{max} \left( { 2 \sqrt{k} \over \beta^* \omega y } , { 500 \nu \over y^2 \omega } \right) \right]^2 \right]](/W/images/math/8/7/d/87d29560b46100813cef49e9f861f1cc.png)
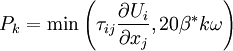
![F_1=\mbox{tanh} \left\{ \left\{ \mbox{min} \left[ \mbox{max} \left( {\sqrt{k} \over \beta ^* \omega y}, {500 \nu \over y^2 \omega} \right) , {4 \sigma_{\omega 2} k \over CD_{k\omega} y^2} \right] \right\} ^4 \right\}](/W/images/math/1/4/3/1438132576e08de1da7394c694172485.png)