Overset grids
From CFD-Wiki
m (→External links) |
|||
| Line 47: | Line 47: | ||
== External links == | == External links == | ||
* [http://www.na.chalmers.se/~andersp/chalmesh/chalmesh.html Chalmesh] | * [http://www.na.chalmers.se/~andersp/chalmesh/chalmesh.html Chalmesh] | ||
| + | * [http://people.nas.nasa.gov/~rogers/cgt/doc/man.html Chimera Grid Tools] | ||
Revision as of 17:03, 21 September 2005
Contents |
Introduction
A common difficulty in simulating complex fluid flow problems is that not every geometry can be well represented using a single, contiguous (structured or unstructured) grid. In many cases, different geometrical features are best represented by different grid types. One approach these difficulties is the construction of a grid system made up of blocks of overlapping structured grids. This technique is referred to as the Chimera or overset grid approach. In a full Chimera grid system, a complex geometry is decomposed into a system of geometrically simple overlapping grids. Boundary information is exchanged between these grids via interpolation of the flow variables, and many gridpoints may not be used in the solution (these points are sometimes called hole points). Each block has boundary or fringe points, which lie in the interior of a neighboring block (or blocks) and will require information from that containing block. The data that must be generated to successfully complete a Chimera-type calculation is not insignificant, and thus has been automated to a high degree. In general terms, there are three steps to setting up an overset simulation:
- Grid generation
- Hole cutting
- Determination of interpolation weights
Note that in some systems, one or more (or all) of these steps may be combined. Finally, while the Chimera technique is most often associated with traditional finite volume/difference CFD codes, it can in principle be applied with other discretization schemes.
Grid generation
Grids for an overset simulation are generally simple and structured, and are often generated hyperbolic or marching techniques. (Add more here)
Hole Cutting
Add example here
Chimera interpolation
Many of the tools required to compute the data required to do an overset simulation can be built upon the trilinear interpolation formula. The discussion here assumes hexahedral cells with nodal representation of flow variables available. Given a hexahedral cell formed by eight points
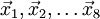(see figure, which needs to be redrawn), the coordinates of any point in the interior of this cell may be written as
(This equation is really ugly)
where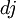 
, 
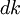, and 
are the interpolation weights and are in
the interval ![[0,1]](/W/images/math/c/c/f/ccfcd347d0bf65dc77afe01a3306a96b.png)
. For any boundary point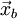 
and any cell base point 
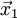we can solve the interpolation for for the weights. If the cell does not contain 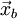, then one or more of the weights will not be in the proper range. This can be used to construct a Newton-like gradient search technique. For more details, the reader is referred to the chapter by Meakin (1999) and the references contained therein. In practice, the initial condition for this solution procedure is quite important. It is usually best to perform some spatial partitioning to narrow the search range.
Software
A number of packages have been developed for this purpose. Two examples are NASA's PEGASUS [Suhs et al 2002], which is primarily concerned with the determination of overset data for existing grids, and Chalmesh [Petersson 1999], which generates grids and the interpolation data simultaneously.
References
Find the references again
