Introduction to turbulence/Wall bounded turbulent flows
From CFD-Wiki
(→The “inner” turbulent boundary layer) |
|||
| Line 295: | Line 295: | ||
<table width="70%"><tr><td> | <table width="70%"><tr><td> | ||
:<math> | :<math> | ||
| - | 0 = - \left\{ \left\langle uv \right\rangle - \left\langle uv \right\rangle | + | 0 = - \left\{ \left\langle uv \right\rangle - \left\langle uv \right\rangle |_{y=0} \right\} + \nu \left\{ \frac{\partial U}{ \partial y} - \frac{\partial U}{ \partial y} \bigg|_{y=0} \right\} |
</math> | </math> | ||
</td><td width="5%">(18)</td></tr></table> | </td><td width="5%">(18)</td></tr></table> | ||
Revision as of 15:37, 29 October 2011
Contents |
Introduction
Without the presence of walls or surfaces, turbulence in the absence of density fluctuations could not exist. This is because it is only at surfaces that vorticity can actually be generated by an on-coming flow is suddenly brought to rest to satisfy the no-slip condition. The vorticity generated at the leading edge can then be diffused, transported and amplified. But it can only be generated at the wall, and then only at the leading edge at that. Once the vorticity has been generated, some flows go on to develop in the absence of walls, like the free shear flows we considered earlier. Other flows remained “attached” to the surface and evolve entirely under the influence of it. These are generally referred to as “wall-bounded flows” or “boundary layer flows”. The most obvious causes for the effects of the wall on the flow arise from the wall-boundary conditions. In particular,
- The kinematic boundary condition demands that the normal velocity of
the fluid on the surface be equal to the normal velocity of the surface. This means there can be no-flow through the surface. Since the velocity normal to the surface cannot just suddenly vanish, the kinematic boundary condition ensures that the normal velocity components in wall-bounded flows are usually much less than in free shear flows. Thus the presence of the wall reduces the entrainment rate. Note that viscosity is not necessary in the equations to satisfy this condition, and it can be met even by solutions to to the inviscid Euler’s equations.
- The no-slip boundary condition demands that the velocity component tangential to the wall be the same as the tangential velocity of the wall. If the
wall is at rest relative, then the no-slip condition demands the tangential flow velocity be identically zero at the surface.
Figure 8.1: Flow around a simple airfoil without separation.
It is the no-slip condition, of course, that led Ludwig Prandtl1 to the whole
idea of a boundary layer in the first place. Professor Prandt literally saved fluid mechanics from d’Alembert’s paradox: the fact that there seemed to be no drag in an inviscid fluid (not counting form drag). Prior to Prandtl, everyone thought that as the Reynolds number increased, the flow should behave more and more like an inviscid fluid. But when there were surfaces, it clearly didn’t. Instead of behaving like those nice potential flow solutions (like around cylinders, for example), the flow not only produced drag, but often separated and produced wakes and other free shear flows. Clearly something was very wrong, and as a result fluid mechanics didn’t get much respect from engineers in the 19th century.
And with good reason: how useful could a bunch of equations be if they couldn’t
find viscous drag, much less predict how much. But Prandtl’s idea of the boundary
layer saved everything.
Prandtl’s great idea was the recognition that the viscous no-slip condition could not be met without somehow retaining at least one viscous stress term in the equations. As we shall see below, this implies that there must be at least two length scales in the flow, unlike the free shear flows we considered in the previous chapter for which the mean flow could be characterized by only a single length scale. The second length scale characterizes changes normal to the wall, and make it clear precisely which viscous term in the instantaneous equations is important.
Review of laminar boundary layers
Let’s work this all out for ourselves by considering what happens if we try to
apply the kinematic and no-slip boundary conditions to obtain solutions of the
Navier-Stokes equations in the infinite Reynolds number limit. Let’s restrict our
attention for the moment to the laminar flow of a uniform stream of speed, 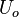 ,
around a body of characteristic dimension, D, as shown in Figure 8.1. It is easy
to see the problem if we non-dimensionalize our equations using the free stream
boundary condition and body dimension. The result is:
,
around a body of characteristic dimension, D, as shown in Figure 8.1. It is easy
to see the problem if we non-dimensionalize our equations using the free stream
boundary condition and body dimension. The result is:
|
| (1) |
where 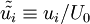 ,
, 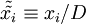 ,
,  and
and 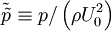 . The kinematic viscosity,
. The kinematic viscosity, 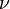 has disappeared entirely, and is included in the Reynolds number defined by:
has disappeared entirely, and is included in the Reynolds number defined by:
|
| (2) |
Now consider what happens as the Reynolds number increases, due to the increase of 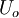 or
or 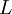 , or even a decrease in the viscosity. Obviously the viscous terms become relatively less important. In fact, if the Reynolds number is large enough it is hard to see at first glance why any viscous term should be retained at all. Certainly in the limit as
, or even a decrease in the viscosity. Obviously the viscous terms become relatively less important. In fact, if the Reynolds number is large enough it is hard to see at first glance why any viscous term should be retained at all. Certainly in the limit as  , our equations must reduce to Euler’s equations which have no viscous terms at all; i.e., in dimensionless form,
, our equations must reduce to Euler’s equations which have no viscous terms at all; i.e., in dimensionless form,
|
| (3) |
Now if we replace the Navier-Stokes equations by Euler’s equations, this presents no problem at all in satisfying the kinematic boundary condition on the body’s surface. We simply solve for the inviscid flow by replacing the boundary of the body by a streamline. This automatically satisfies the kinematic boundary condition. If the flow can be assumed irrotational, then the problem reduces to a solution of Laplace’s equation, and powerful potential flow methods can be used.
In fact, for potential flow, it is possible to show that the flow is entirely determined by the normal velocity at the surface. And this is, of course, the source of our problem. There is no role left for the viscous no-slip boundary condition. And indeed, the potential flow has a tangential velocity along the surface streamline that is not zero. The problem, of course, is the absence of viscous terms in the Euler equations we used. Without viscous stresses acting near the wall to retard the flow, the solution cannot adjust itself to zero velocity at the wall. But how can viscosity enter the equations when our order-of-magnitude analysis says they are negligible at large Reynolds number, and exactly zero in the infinite Reynolds number limit. At the end of the nineteenth century, this was arguably the most serious problem confronting fluid mechanics.
Prandtl was the first to realize that there must be at least one viscous term
in the problem to satisfy the no-slip condition. Therefore he postulated that the
strain rate very near the surface would become as large as necessary to compensate for the vanishing effect of viscosity, so that at least one viscous term remained. This very thin region near the wall became known as Prandtl’s boundary layer, and the length scale characterizing the necessary gradient in velocity became known as the boundary layer “thickness”.
Prandtl’s argument for a laminar boundary layer can be quickly summarized using the same kind of order-of-magnitude analysis we used in Chapter 7 (here necessary make navigation !!!). For the leading viscous term:
|
| (4) |
where 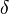 is the new length scale characterizing changes normal to the plate near the wall and we have assumed
is the new length scale characterizing changes normal to the plate near the wall and we have assumed 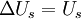 . In fact, for a boundary layer next to walls driven by an external stream speed
. In fact, for a boundary layer next to walls driven by an external stream speed  . For the leading convection term:
. For the leading convection term:
|
| (5) |
The viscous term can survive only if it is the same order of magnitude as the convection term. Hence it follows that we must have:
|
| (6) |
This in turn requires that new length scale 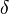 must satisfy:
must satisfy:
|
| (7) |
or
|
| (8) |
Thus, for a laminar flow 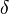 grows like
grows like  . Now if you go back to your fluid mechanics texts and look at the similarity solution for a Blasius boundary layer, you will see this is exactly right if you take
. Now if you go back to your fluid mechanics texts and look at the similarity solution for a Blasius boundary layer, you will see this is exactly right if you take 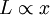 , which is what we might have guessed anyway.
, which is what we might have guessed anyway.
It is very important to remember that the momentum equation is a vector equation, and we therefore have to scale all components of this vector equation the same way to preserve its direction. Therefore we must carry out the same kind of estimates for the cross-stream momentum equations as well. For a laminar boundary layer this can be easily be shown to reduce to:
|
| (9) |
Note that a only single term survives in the limit as the Reynolds number goes to
infinity, the cross-stream pressure gradient. Hence, for very large Reynolds number, the pressure gradient across the boundary layer equation is very small. Thus the pressure in the boundary is imposed on it by the flow outside the boundary layer. And this flow outside the boundary layer is governed to first order by Euler’s equation.
The fact that the pressure is imposed on the boundary layer provides us an
easy way to calculate such a flow. First calculate the inviscid flow along the
surface using Euler’s equation. Then use the pressure along the surface from this inviscid solution together with the boundary layer equation to calculate the boundary layer flow. If you wish, you can even use an iterative procedure where you recalculate the outside flow over a streamline which was been displaced from the body by the boundary layer displacement thickness, and then re-calculate the boundary layer, etc. Before modern computers, this was the only way to calculate the flow around an airfoil, for example.
The "outer" turbulent boundary layer
The understanding of turbulent boundary layers begins with exactly the same averaged equations we used for the free shear layers of Chapter 7(here necessary to add link!!!); namely,
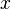 -component:
-component:
|
| (10) |
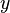 -component:
-component:
|
| (11) |
two-dimensional mean continuity
|
| (12) |
In fact, the order of magnitude analysis of the terms in this equation proceeds exactly the same as for free shear flows. If we take  , then the ultimate problem again reduces to how to keep a turbulence term. And, as before this requires:
, then the ultimate problem again reduces to how to keep a turbulence term. And, as before this requires:
|
| (00) |
No problem, you say, we expected this. But the problem is that by requiring this be true, we also end up concluding that even the leading viscous term is also negligible! Recall that the leading viscous term is of order:
|
| (13) |
compared to the unity.
In fact to leading order, there are no viscous terms in either component of the
momentum equation. In the limit as 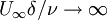 , they are exactly the same as for the free shear flows we considered earlier; namely,
, they are exactly the same as for the free shear flows we considered earlier; namely,
|
| (14) |
and
|
| (15) |
And like the free shear flows these can be integrated from the free stream to a
given value of 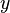 to obtain a single equation:
to obtain a single equation:
|
| (16) |
The last term in brackets is the gradient of the difference in the normal Reynolds stresses, and is of order 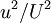 compared to the others, so is usually just ignored. The bottom line here is that even though we have attempted to carry out an order of magnitude analysis for a boundary layer, we have ended up with exactly the equations for a free shear layer. Only the boundary conditions are different— most notably the kinematic and no-slip conditions at the wall. Obviously, even though we have equations that describe a turbulent boundary layer, we cannot satisfy the no-slip condition without a viscous term. In other words, we are right back where we were before Prandtl invented the boundary layer for laminar flow! We need a boundary layer within the boundary layer to satisfy the no-slip condition. In the next section we shall in fact show that such an inner boundary layer exists. And that everything we analysed in this section applies only to the outer boundary layer — which is NOT to be confused with the outer flow which is non-turbulent and still governed by Euler’s equation. Note that the presence of
compared to the others, so is usually just ignored. The bottom line here is that even though we have attempted to carry out an order of magnitude analysis for a boundary layer, we have ended up with exactly the equations for a free shear layer. Only the boundary conditions are different— most notably the kinematic and no-slip conditions at the wall. Obviously, even though we have equations that describe a turbulent boundary layer, we cannot satisfy the no-slip condition without a viscous term. In other words, we are right back where we were before Prandtl invented the boundary layer for laminar flow! We need a boundary layer within the boundary layer to satisfy the no-slip condition. In the next section we shall in fact show that such an inner boundary layer exists. And that everything we analysed in this section applies only to the outer boundary layer — which is NOT to be confused with the outer flow which is non-turbulent and still governed by Euler’s equation. Note that the presence of 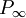 in our outer boundary equations means that (to first order in the turbulence intensity), the pressure is still imposed on the boundary layer by the flow outside it, exactly as for laminar boundary layers (and all free shear flows, for that matter).
in our outer boundary equations means that (to first order in the turbulence intensity), the pressure is still imposed on the boundary layer by the flow outside it, exactly as for laminar boundary layers (and all free shear flows, for that matter).
The “inner” turbulent boundary layer
We know that we cannot satisfy the no-slip condition unless we can figure out
how to keep a viscous term in the governing equations. And we know there can
be such a term only if the mean velocity near the wall changes rapidly enough so
that it remains, no matter how small the viscosity becomes. In other words, we
need a length scale for changes in the 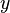 -direction very near the wall which enables us keep a viscous term in our equations. This new length scale, let’s call it
-direction very near the wall which enables us keep a viscous term in our equations. This new length scale, let’s call it  , is going to be much smaller than
, is going to be much smaller than 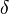 , the boundary layer thickness. But how much smaller?
, the boundary layer thickness. But how much smaller?
Obviously we need to go back and look at the full equations again, and re-scale
them for the near wall region. To do this, we need to first decide how the mean
and turbulence velocities scale near the wall scale. We are clearly so close to the wall and the velocity has dropped so much (because of the no-slip condition) that it makes no sense to characterize anything by  . But we don’t have anyway of knowing yet what this scale should be, so let’s just call it
. But we don’t have anyway of knowing yet what this scale should be, so let’s just call it 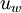 and define it later. Also, we do know from experiment that the turbulence intensity near the wall is relatively high (30% or more). So there is no point in distinguishing between a turbulence scale and the mean velocity, we can just use
and define it later. Also, we do know from experiment that the turbulence intensity near the wall is relatively high (30% or more). So there is no point in distinguishing between a turbulence scale and the mean velocity, we can just use 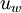 for both. Finally we will still use
for both. Finally we will still use 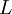 to characterize changes in the
to characterize changes in the 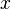 -direction, since these will vary no more rapidly than in the outer boundary layer above this very near wall region we are interested in.
-direction, since these will vary no more rapidly than in the outer boundary layer above this very near wall region we are interested in.
For the complete 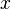 -momentum equation we estimate
-momentum equation we estimate
|
| (00) |
|
| (00) |
where we have used the continuity equation to estimate 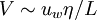 near the wall.
near the wall.
Now as always, we have to decide which terms we have to keep so we know what to compare the others with. But that is easy here, we MUST insist that at least one viscous term survive. Since the largest is of order 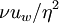 , we can divide by it to obtain:
, we can divide by it to obtain:
|
| (00) |
|
| (00) |
Now we have an interesting problem. We have the power to whether the Reynolds shear stress term survives or not by our choice of 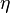 ; i.e. we can pick
; i.e. we can pick 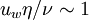 or
or  . Note that we can not choose it so this term blows up, or else our viscous term will not be at least equal to the leading term. The most general choice is to pick
. Note that we can not choose it so this term blows up, or else our viscous term will not be at least equal to the leading term. The most general choice is to pick  so the Reynolds shear stress remains too. (This is called the distinguished limit in asymptotic analysis.) By making this choice we eliminate the necessity of having to go back and find there is another layer in which only the Reynolds stress survives — as we shall see below. Obviously if we choose
so the Reynolds shear stress remains too. (This is called the distinguished limit in asymptotic analysis.) By making this choice we eliminate the necessity of having to go back and find there is another layer in which only the Reynolds stress survives — as we shall see below. Obviously if we choose  , then all the other terms vanish, except for the viscous one. In fact, if we apply the same kind of analysis to the
, then all the other terms vanish, except for the viscous one. In fact, if we apply the same kind of analysis to the 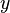 -mean momentum equation, we can show that the pressure in our near wall layer is also imposed from the outside. Moreover, even the streamwise pressure gradient disappears in the limit as
-mean momentum equation, we can show that the pressure in our near wall layer is also imposed from the outside. Moreover, even the streamwise pressure gradient disappears in the limit as 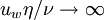 . These are relatively easy to show and left as exercises.
. These are relatively easy to show and left as exercises.
So to first order in  , our mean momentum equation for the near wall region reduces to:
, our mean momentum equation for the near wall region reduces to:
|
| (17) |
In fact, this equation is exact in the limit as 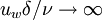 , but only for the very near wall region!
, but only for the very near wall region!
Equation (17) can be integrated from the wall to location 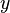 to obtain:
to obtain:
|
| (18) |

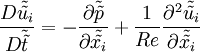
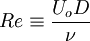
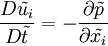

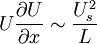
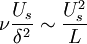
![\delta \sim \left[ \frac{\nu L}{U_{s}} \right]^{1/2}](/W/images/math/d/c/d/dcd40e1c6fe0b947b08a89273c8922a6.png)
![\frac{\delta}{L} \sim \left[ \frac{\nu }{U_{s} L} \right]^{1/2}](/W/images/math/5/1/0/5109f2a3964f3b5997eeeee387ad7f8c.png)
![\left[ \frac{\delta}{L} \right] \left\{ \frac{ \partial \tilde{\tilde{ u}}}{ \partial \tilde{\tilde{t}}} + \tilde{\tilde{u}} \frac{\partial \tilde{\tilde{ u}} }{\tilde{\tilde{ x}}}+ \tilde{\tilde{v}} \frac{\partial \tilde{\tilde{ v}} }{\tilde{\tilde{ y}}} \right\} = - \frac{ \partial \tilde{\tilde{p}}_{\infty}}{ \partial \tilde{\tilde{ y}} } + \frac{1}{Re} \left[ \frac{\delta}{L} \right] \left\{ \frac{ \partial^{2} \tilde{\tilde{v}} }{ \partial \tilde{\tilde{ y}}^{2}} \right\} + \frac{1}{Re} \left\{ \frac{ \partial^{2} \tilde{\tilde{v}} }{ \partial \tilde{\tilde{ x}}^{2}} \right\}](/W/images/math/1/d/8/1d809934e9ae0d115665576eb95a0b1c.png)

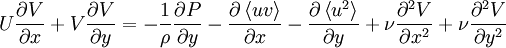
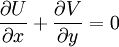
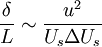

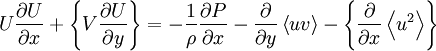

![U \frac{\partial U}{ \partial x} + V \frac{\partial U}{ \partial y} = - \frac{d P_{\infty}}{dx} - \frac{\partial }{ \partial y} \left\langle uv \right\rangle - \left\{ \frac{\partial}{\partial x} \left[ \left\langle u^{2} \right\rangle - \left\langle v^{2} \right\rangle \right] \right\}](/W/images/math/3/d/d/3ddd8fc067d2b2c5ee66fc50ba0343fc.png)
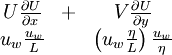

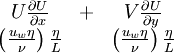
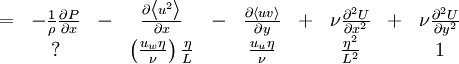
![0 \approx \frac{\partial}{\partial y} \left[ - \left\langle uv \right\rangle + \nu \frac{\partial U}{ \partial y} \right]](/W/images/math/4/9/8/4988d861e7738f9b1f65330d845e58ca.png)
